论文和技术资料

Studies of poroelastic systems increasingly utilize numerical modeling. Especially for geotechnical applications models are set up as a tool to understand phenomena in porous media that deform due to changes of the hydraulic regime, or in which the flow field is affected by changes of the stress regime. Major application fields are waste injection, CO2 injection and steam assisted gravity drainage. Stress and hydraulics are linked in a two-way coupling, also referred to as HM coupling.

If gravity as outer force is considered the common hydrostatic state, showing linear pressure increase with depth, does not match with the zero deformation for the geomechanical force equilibrium equation. Starting from the fundamental descriptions of poroelastic systems with HM coupling the analytical solution for the geostatic state under influence of gravity is derived. The hydrostatic state coincides with strain changing with depth following a quadratic regime.

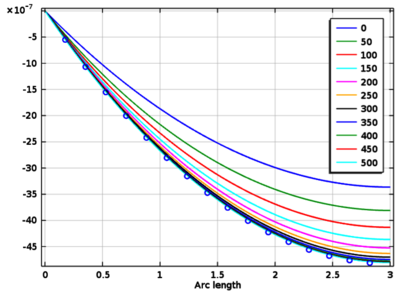
The derived solution is valid for a homogeneous poroelastic system with constant parameters. Using COMSOL Multiphysics® it is shown that numerical and analytical solutions coincide. We use the Geomechanics Module of the COMSOL product suite. Simulations of the transient development show solutions converging to the analytical solution, as shown in the figure. Vertical displacement is shown on the y-axis against height above base on the x-axis. Negative values indicate deformation in direction of gravity. Starting from zero at the fixed base of the deforming layer the absolute values of deformation increase with the arc length. The legend depicts time instants, for which curves are depicted. Dots indicate the analytical solution.

The solution delivers a formula for the vertical strain, and thus for the shrinking of a hypothetical system that was originally not exposed to gravity under the influence of the gravity force. From this we develop the solution for the inverse problem: what is the thickness of a system that is not exposed to an outer force, when the thickness of the system under gravity regime is given. The derived solution can be beneficial for numerical modeling of poroelastic systems under the influence of gravity. The analytical solution can be used as initial condition. Thus simulations of transient developments do not require an artificial initialization phase to obtain an approximation of the steady state. Also a pre-run for direct solution of the steady state, as alternative to transient initialization, becomes obsolete.

Moreover the analytical steady state solution can be used as a reference to compare transient deformations during the simulation. Such multi-purpose utilization in numerical modeling is outlined in a demonstration case that is modeled using COMSOL Multiphysics®. The analytical solution simplifies numerical simulations of poroelastic systems.