Influence of dielectric constant on low-moisture food pasteurisation in a 915 MHz microwave cavity
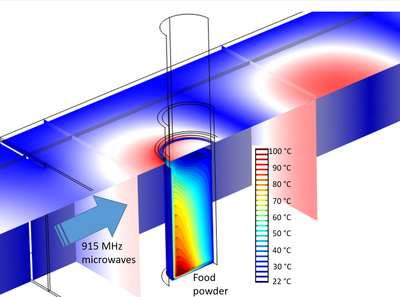
The contamination of food products with low water contents is a safety issue that cannot be disregarded. Despite their low water activity, they are indeed susceptible to infection by micro-organisms that are highly resistant to heat. In this case, conventional pasteurisation techniques (steam or hot air) may prove ineffective (due to low thermal conductivities of food). Moreover, these powdered or particulate products are also often vulnerable to the proliferation of insects. That’s why microwave heating can be a valuable alternative to conventional treatments, especially if 915 MHz is used because of their higher penetration depth. However, optimizing temperature distribution in food is essential for guaranteeing treatment. Simulation is therefore a relevant tool because it provides access to the temperature and microwave fields throughout the product. The energy due to microwaves depends on the electric field and the dielectric properties of the food. These ones are defined by the complex permittivity "ε" _"r" ^"*" ("ε" _"r" ^"*" "= " "ε" _"r" ^"'" "- j∙" "ε" _"r" ^"''" " ; " "j" ^"2" " = -1)" and characterize the interaction of a material with electromagnetic fields. The first, named the dielectric constant, reflects the ability of a material to store energy and the second, the loss factor, represents the conversion of electromagnetic energy into heat. In the case of products with low water contents, these are difficult to measure and give rise to some uncertainties (Frabetti et al., 2023). The aim of this work is to show the influence of measurement uncertainties for these properties on temperature fields. For this purpose, numerical simulations using COMSOL Multiphysics® 6.2 were carried out. The Maxwell’s equations were solved in an optimized resonant cavity using the RF module for an incident power of 200 W to determine the electric field distribution (figure 1). The Heat Transfer module was used for the heating of 80 g of paprika powder in a quartz tube of 4 mm of thickness, 240 mm height and 46 mm intern diameter. To simplify the problem, heat transfer equations were solved only in the powder and the quartz tube and natural convection was used as boundary conditions. Previous simulations have shown that a constant value of "ε" _"r" ^"''" is unable to predict the temperature increase in the sample and a linear relationship between the loss factor and the temperature is required. Based on experimental values of "ε" _"r" ^"''" at ambient temperature (Frabetti et al., 2023), this relationship can take the following form: "ε" _"r" ^"''" " = a T + b" with a = 0.01275 K^"-1" and b = -3.538 or -3.498 for 293 K < T < 393 K. Regarding the dielectric constant, this one was measured at 1.53 with the open-ended coaxial probe and at 1.96 with the cavity perturbation method. Simulations were carried out with several pairs of values ("ε" _"r" ^"'" , b) and results concerning the temperature at the centre of the paprika powder are shown in figure 2. It appears from the temperature rise that, for these specific dielectric properties, uncertainties in the dielectric constant measurement ("ε" _"r" ^"'" ), give rise to greater temperature differences than those in the loss factor.
下载
- rouaud_10231_poster.pdf- 0.57MB
